Circular sector

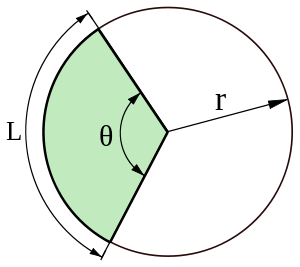
A circular sector is shaded in green

A circular sector or circle sector (symbol: ⌔), is the portion of a disk enclosed by two radii and an arc, where the smaller area is known as the minor sector and the larger being the major sector. In the diagram, θ is the central angle in radians, $r$ the radius of the circle, and $L$ is the arc length of the minor sector.

A sector with the central angle of 180° is called a half-disk and is bounded by a diameter and a semicircle. Sectors with other central angles are sometimes given special names, these include quadrants (90°), sextants (60°) and octants (45°), which come from the sector being one 4th or 6th or 8th part of a full circle, respectively.

The angle formed by connecting the endpoints of the arc to any point on the circumference that is not in the sector is equal to half the central angle.

Area

The total area of a circle is $π$ r². The area of the sector can be obtained by multiplying the circle's area by the ratio of the angle and 2 $π$ (because the area of the sector is proportional to its angle, and 2 $π$ is the angle for the whole circle, in radians):

A=\pi r^{2}\cdot {\frac {\theta }{2\pi }}={\frac {r^{2}\theta }{2}}

The area of a sector in terms of L can be obtained by multiplying the total area $π$ r² by the ratio of L to the total perimeter 2 $π$ r.

A=\pi r^{2}\cdot {\frac {L}{2\pi r}}={\frac {r\cdot L}{2}}

Another approach is to consider this area as the result of the following integral :

A=\int _{0}^{\theta }\int _{0}^{r}dS=\int _{0}^{\theta }\int _{0}^{r}{\tilde {r}}\,d{\tilde {r}}\,d{\tilde {\theta }}=\int _{0}^{\theta }{\frac {1}{2}}r^{2}\,d{\tilde {\theta }}={\frac {r^{2}\theta }{2}}

Converting the central angle into degrees gives

A=\pi r^{2}\cdot {\frac {\theta ^{\circ }}{360^{\circ }}}

Arc length

The length L of the arc of a sector is given by

L=\theta \cdot r,

where θ is in radians.

If the angle is given in degrees, then

L=\theta ^{\circ }\cdot r\cdot {\frac {\pi }{180^{\circ }}}

Perimeter

The length of the perimeter of a sector is the sum of the arc length and the two radii:

P=L+2r=\theta r+2r=r(\theta +2)

where θ is in radians.

References

Gerard, L. J. V. The Elements of Geometry, in Eight Books; or, First Step in Applied Logic, London, Longman's Green, Reader & Dyer, 1874. p. 285

External links

Definition and properties of a circle sector with interactive animation
Weisstein, Eric W. "Circular sector". MathWorld.

This article is issued from Wikipedia - version of the 10/28/2016. The text is available under the Creative Commons Attribution/Share Alike but additional terms may apply for the media files.