Armadillo projection
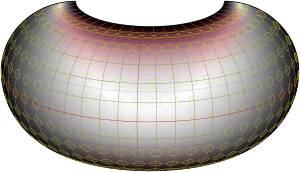
The armadillo projection is a map projection used for world maps. It is neither conformal nor equal-area but instead affords a view evoking a perspective projection while showing most of the globe instead of the half or less that a perspective would. The projection was presented in 1943 by Erwin Raisz (1893–1968) as part of a series of "orthoapsidal" projections, which are perspectives of the globe projected onto various surfaces. This one in the series has the globe projected onto half a torus. Raisz singled it out and named it the "armadillo" projection.[1]
The toroidal shape and the angle it is viewed from tend to emphasize continental areas by eliminating or foreshortening swaths of ocean. In the pure case of projecting the half-torus, New Zealand cannot be seen, as in the images here. However, in publications, the projection often develops a "pigtail" which shows the rest of Australia as well as New Zealand.
Raisz coined the term orthoapsidal as a combination of orthographic and apsidal. He used it to mean drawing a parallel-meridian network, or graticule, on any suitable solid other than a sphere, and then making an orthographic projection of that.[2]
Formulas
Given a radius of sphere R, central meridian λ0 and a point with geographical latitude φ and longitude λ, plane coordinates x and y can be computed using the following formulas:[3]
In this formulation, no latitude more southerly than φs should be plotted for the given longitude. The y-axis coincides with the central meridian.
See also
References
- ↑ Snyder, John P. (1993). Flattening the Earth: 2000 Years of Map Projections. Chicago: University of Chicago Press. p. 267–268.
- ↑ Raisz, Erwin J. (1962). Principle of Geography. New York: McGraw-Hill. p. 181.
- ↑ Snyder, John P. (1989). An Album of Map Projections. Professional Paper 1453. Denver: USGS. p. 238. ISBN 978-0160033681. Retrieved 2014-09-27.
External links
- Description and characteristics at mapthematics.com